Solution to Exercise 2.57.
The
value of
—![]() Bertrand Russell (1872–1970)
Bertrand Russell (1872–1970)
Exercise
2.57. Prove Theorem 2.12. Given a line and a point not on the line,
there exists a unique line perpendicular to the given line through the given
point.
Proof. Let l be a line and P be a point not on line l.
Let A
and B be two points on line l. By the Angle Construction Postulate,
there is a ray AQ with Q and P on opposites sides of line l
and By the Ruler Postulate, there is a point R on line AQ and on the same side of line l
as Q such that AR = AP. Note that P and R are on opposite sides of line l.
Hence, by the Plane Separation Postulate, there is a point C on line l such that P-C-R. One of the following is true: A-B-C, C = B, A-C-B, C = A, or C-A-B.
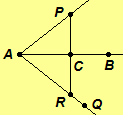
Case 1. Assume A-B-C, C = B, or A-C-B.
Since
and
we have
Hence,
Thus
and
are a linear pair of congruent angles, since P-C-R.
 Since
a linear pair of congruent angles are right angles,
line PR is perpendicular to line
Since
a linear pair of congruent angles are right angles,
line PR is perpendicular to line
Case 2. Assume C = A. Since and P-A-R,
and
are a linear pair of congruent angles. Since a linear pair of congruent angles are right angles, line PR is perpendicular to line
Case 3. Assume C-A-B. Then and
are a linear pair. Also,
and
are a linear pair. Hence, by the Supplement
Postulate and
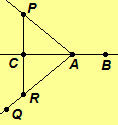 the definition of supplementary angles,
the definition of supplementary angles, and
Therefore,
Since
and
we have
Hence,
Thus
and
are a linear pair of congruent angles, since P-C-R. Since a linear
pair of congruent angles are right angles,
line PR is perpendicular to line
All cases show that there exists a line
through P perpendicular to line l. We need to show that the line is
unique. Suppose there are two lines through P
that are perpendicular to line l. Let
A and B be the points on line l where
the two perpendicular lines intersect line l.
Let C be a point on line l such that A-B-C. Then is an exterior angle of
.
By the Exterior Angle Theorem,
Since line PB
and line PA are perpendicular to line
l,
and
are right angles. Thus,
But, this is a contradiction. Therefore, the
line through P that is perpendicular
to line l is unique.//