Chapter three is the first of five chapters developing a model of the macro economy which analyzes long run performance based on full employment (classical assumption).

A. Labor Market (chapter 3)

B. Goods Market (chapter 4)

C. Assets Market (chapter 7)

I. The Production Function: (Section 3.1) Shows how much output the economy produces with given amounts of labor and capital.

A. Factors of production: What is used in the production process?

1. Capital
2. Labor
3. Other (raw materials, land energy…)
4. Productivity of factors depends on technology and management productivity refers to the return received from inputs, if there is an increase in productivity each unit of input (Capital, Labor or other) will produce more output.

B. Production function: A mathematical expression relating the amount of output produced to the quantities of capital and labor used.

Y=AF(K,N)

Where: Y= real output in a given time period

A= a measure of productivity

K= the capital stock used in the period

N= the number of workers employed ( the labor input)

F= a function relating Y to K and N

Example:

Y=A(K^0.5N ^0.5) Note: A variable raised to the 0.5 power is the same as taking the square root of the variable (Section A.6 of Appendix A)

Let K = 2500, n = 9000, A =2 then output would be equal to:

Y = 2(2500^.05900^0.5) =2(50*30) =2 (1500) = 3000

What if productivity doubles to 4? Then output would increase to 6000.

What would cause this to happen?

C. Above is an example of a Cobb Douglas production function Y= A K^aN^1-a good approximation for the US economy is when a is equal to 0.3

D. The shape of the production function:

Easiest way to graph the production function is by keeping the measure of productivity and amount of one of the factors (capital or labor) constant, allowing the other to vary. For now assume that the amount of labor is held constant and capital is allowed to vary.

a. The production function slopes upward from left to right

b. The slope of the production function becomes flatter form left to right.

E. The marginal productivity of capital: How much does an additional unit of capital increase output? Will it be equal to the slope of the lint tangent to the production function at a particular point.

a. The marginal productivity of capital will always be positive.

b. Diminishing marginal productivity of capital: (each additional unit will result in a smaller increase in output)

F. Could also keep capital fixed and productivity fixed and graph a production function relating output and labor input. Will find marginal productivity of labor just as we found marginal productivity of capital.

a. The marginal productivity of labor will always be positive.

b. Diminishing marginal productivity of labor.

G. Supply Shocks

A change in the amount of output which can be produced for a given amount of labor and capital (also termed a productivity shock)

a. Positive supply shock causes the slope of the production function to increase at every level of output (the production function shifts upward).

b. Negative supply shock causes the slope of the production function to decrease at every level of output (the production function shifts downward).

II. The Demand for Labor: (Section 3.2) How Much labor so firms demand?

Note: we are going to discuss the demand for the labor supply to labor the combine them to find the equilibrium amount of labor used – when this occurs we will assume that the classical assumptions hold and that wages and prices will adjust quickly to bring about equilibrium

A. Assumptions concerning the labor market:

a. Workers are all alike

b. The labor market is competitive (Know what is meant by competitive) c. Firms act to maximize profits.

B. The marginal product of labor and labor demand.

a. The marginal revenue product of labor (MRPN) MRPN is the additional revenue received from adding another worker. MRPN =- price x MPN

b. The cost of hiring the additional labor is the wage rate paid to the employee. The nominal wage is the actual amount paid to the worker. You could also look at the wage rate in "real" terms. In this case it represents the amount of output it would cost you to pay the worker.

Real wage = Nominal Wage/Price  (Most of the time we will assume that the price = 1)

Real wage = MPN is the same as nominal wage = MRPN

c. To decide how much labor to hire you will compare the cost of hiring an additional worker (Nominal wage) to the benefit of hiring he additional worker (MRPN)

If nominal wage > MRPN then the firm should decrease the demand of labor starting form equilibrium Nominal Wage = MRPN if the nominal wage increases, then Nominal Wage > MRPN. The firm will decrease its demand for labor since the last worker(s) are not producing marginal revenue to cover their nominal wage.

If nominal wage< MRPN then the firm should increase the demand for labor staring from equilibrium Nominal Wage = MRPN if the nominal wage decreases, then Nominal Wage < MRPN. The firm will increase its demand for labor since additional worker(s) can be added who will produce marginal revenue product which is greater than their wage.

C. Costs and benefits of hiring and extra worker can also be expressed in terms of eal wage and MPN (real wage = p x nominal wage)

a. If real wage > the marginal productivity of Labor (MPN) the firm is paying more than the worker produces and should reduce the amount of workers (nominal wage < MRPN)

b. If real wage < MPN the firm is paying an amount less than the worker produces, adding another worker will increase profit so the firm should increase that amount of workers (nominal wage >MRPN)

c. The firm will maximize profits when the real wage is equal to MPN.

D. The marginal product of labor and the labor demand curve.

a. Relationship between the real wage (vertical axis) and the amount of Labor demanded (horizontal axis).

b. Relationship between MPN and real wage.

c. Labor demand is downward sloping: firms will hire less labor the higher the real wage.

E. Factors that shift the labor demand curve.

a. A change in the real wage causes a movement along the demand curve not a shift in the demand curve.

b. Adverse supply shock will decrease MPN therefore the labor demand curve will shift to the left. Opposite for beneficial supply stock.

c. An increase in the capital stock increases MPN so the labor demand curve shifts right.

F. Aggregate Labor Demand Curve

a. Sum of all individual firms labor demand

b. Shifts in the labor demand curve are caused by the same factors that shift an individual labor demand curve.

A. Supply of labor is determined by individual decision making.

1. Aggregate Supply is the sum of individuals’ labor supply.

2. The tradeoff between labor and leisure will determine the individuals choice about the amount of labor supplied.

B. The labor supply curve

1. The labor supply curve relates quantity of labor supplied to the real wage.

2. An increase in the real wage should increase the quantity of labor supplied. Therefore the labor supply curve will be upward sloping. (higher wage will encourage more work).

C. Factors that shift the labor supply curve

1. Increase in wealth will reduce labor supply – increase future expected earnings will reduce labor supply.

D. Aggregate labor supply

1. Increase in current real wage causes individuals to work more and new people to enter the labor force. Therefore the labor supply curve also slopes upward.

2. Factors which increase the amount of labor supplied

a. Decrease in wealth

b. Decrease in future real wage

c. Increase in working-age population

d. Increase in labor force participation

A. Equilibrium when Labor Demand equals Labor Supply

1. Classical model of labor market – wages adjust quickly to bring the market to equilibrium

2. Equilibrium determines the full employment level of employment and the market-clearing real wage.

3. Factors that shift the labor supply or labor demand curve affect the full employment level of employment and market-clearing real wage.

4. Problem with classical model – does not explain unemployment

B. Full employment output

1. 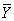= AF (K, )

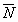2. full employment output is affected by changes in the full employment level of employment or changes in the production function.

C. Applications  (MAY BE A GOOD IDEA TO PRACTICE THESE)

1. Oil Price shocks

2. Technical change