1.     Let us explore the Cobb-Douglas production function introduced in class.  Although not explicitly stated, the growth equation listed in 6.1—The Sources of Growth is this Cobb-Douglas format.  Let us look at an economy over twenty years.  Its total output has grown from 6,501 to 12,679, its capital stock has risen from 22,033 to 38,440, and its labor force has increased from .107 to .143.  All measurements are in real terms.  Calculate the contributions to economic growth of growth in capital, labor, and productivity:

a.     Assuming that α= 0.3 and β = 0.7.

b.     Assuming that α= 0.5 and β= 0.5.

The Cobb-Douglas production function is a very common function used by economists and the .3/.7 constant returns to scale fits the US economy well.  The numbers used in the problem are the actual US figures for 1980, 1990, and 2000 in trillions.

growth in output (12,679-6,501) / 6,501 = 95.03%

growth in capital stock (38,440-22,033) / 22,033 = 74.47%

growth in the labor force (this is actually employed workers)  (.143-.107) / .107 = 33.64%

DA/A = DY/Y - a_K DK/K - a_N DN/N

This is 49.14% in part a and 40.98% in part b.

2.     For a particular economy, the following capital input K and labor input N were reported in four different years:

The production function in this economy is

Y = K^.3N^.7    where Y is total output.

a.     Find total output, the capital-labor ratio, and output per worker in each year. Compare year 1 with year 3 and year 2 with year 4.  Can this production function be written in per-worker form?  If so, write algebraically the per-worker form of the production function.

b.     Repeat Part (a) but assume now that the production function is Y = K^.3N^.8   

As in the previous problem, these are real numbers, but this is a very restrictive production function.  Without a total factor productivity term the numbers are way off. 

3.     An economy has the per-worker production function

 y_t = 3k^0.5

where is output per worker and is the capital-labor ratio. The depreciation rate is 0.08, and the population growth rate is 0.04. Saving is  S_t = 0.1Y_t  where is total national saving and is total output.

a.     What are the steady-state values of the capital-labor ratio, output per worker, and consumption per worker?

sf(k) = (n + d)k

      0.1 ´ 3k^.5 = (0.08 + 0.04)k

      0.3k^.5 = 0.12k

      0.3/0.12 = k/k^.5

      2.5 = k^.5

      k = 6² = 6.25

      y = 3k^.5 =  7.5

      c = y - (n + d)k = 6.75

The rest of the problem shows the effects of changes in the three fundamental determinants of long-run living standards.

b.     Repeat Part (a) for a saving rate of 0.12 instead of 0.1.

sf(k) = (n + d)k

      0.12 ´ 3k^.5 = (0.08 + 0.04)k

      3 = k^.5

      k = 3² = 9

      y = 3k^.5 = 9

      c = y - (n + d)k = 7.92

c.     Repeat Part (a) for a population growth rate of 0.06 (with a saving rate of 0.1).

sf(k) = (n + d)k

      0.1 ´ 3k^.5 = (0.08 + 0.06)k

      2.1428 = k^.5

      k = 4.59

      y = 6.429

      c = y - (n + d)k = 5.786

d.     Repeat Part (a) for a production function of y_t = 4k^0.5

Assume that the saving rate and population growth rate are at their original  values.

sf(k) = (n + d)k

      0.1 ´ 4k^.5 = (0.08 + 0.04)k

      k = 11.11

      y = 13.33

      c = y - (n + d)k = 12

4.     According to our growth model, how would each of the following affect output per worker, investment per worker, consumption per worker, and the capital to labor ratio in the long run (that is, in the steady state)?  Illustrate with a graph!

a.     A hurricane destroys a portion of the nation’s capital stock.

             The destruction of some of a country’s capital stock would have no effect on the final steady state, because there has been no change in s, f, n, or d. Instead, k is reduced temporarily, but equilibrium forces eventually drive k to the same steady-state value as before.

b.     Congress decides to allow anyone to migrate to the United States.  As most of the new population has a higher birth rate compared to the current population, the overall population growth rate increases.

Immigration raises n from n¹ to n² . The rise in n lowers steady-state k, leading to a lower steady-state consumption per worker.

c.     Congress requires all states increase the use non-fossil fuel energy to help protect the environment.  This causes an increase in energy prices.

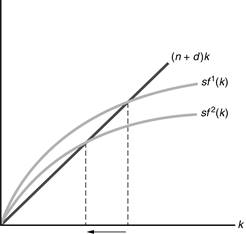
      The rise in energy prices reduces the productivity of capital per worker. This causes both the per-capital production function and sf(k) to shift down. The graph below only illustrates the per-capita investment shift to show the decline in steady-state k. All factors will be reduced.  When you graph this be sure to include the shift of both curves.  I did not have a beautiful pre-made graph so I substituted with this partial , but neat, graph.

d.     Americans become increasing anxious about global warming and increase their saving (a rise in the saving rate) to provide some protection from future problems.

      The changes will look like the graph above, but will  not have a change in the per-capita production function. We know that k and y will increase, but c is dependent upon the golden rule.

e.     Psychologists argue that children need greater parental support to have a well-adjusted childhood.  This cause a large number of two-income families to switch to a single-income family with a stay-at-home parent.  This will cause a permanent decrease in the fraction of the population in the labor force (the population growth rate is unchanged).

      The increase in the size of the labor force does not affect the growth rate of the labor force, so there is no impact on the steady-state capital-labor ratio or on consumption per worker. However, our model has limitations.  We have no way to account for a group that does not participate in production and like all economic models our strength is the cet.par. condition which masks the myriad of effect that will happen in this situation.

5.     An economy has a per-capita production function y = Ak^αh^1-α, where A and a are fixed parameters, y is per-worker output, k is the capital-labor ratio, and h is human capital per worker, a measure of the skills and raining of the average worker. The production function implies that, for a given capital-labor ratio, increases in average human capital raise output per worker.

The economy’s saving rate is s, and all saving is used to create physical capital, which depreciates at rate d. Workers acquire skills on the job by working with capital; the more capital with which they have to work, the more skills they acquire.  We capture this idea by assuming that human capital per worker is always proportional to the amount of physical capital per worker, or h = Bk, where B is a fixed parameter.

  Find the long-run growth rates of physical capital, human capital, and output in the economy.

 I placed this problem on for two reasons.  First, it required an analytical mathematical prowess  that was different from the basic algebra of above.  Second, it shows an expansion of our model.  It is now more realistic , but also more complex.  A tradeoff in economics.  We will talk about this after the test and you will not see this our the last section of chapter 6 on our test.

Assume there are a constant number of workers, N, so that Ny = Y and Nk = K. Since y = Ak^ah^1–a and
h = Bk, then y = Ak^a(Bk)^1–a = (AB^1–a)k. Then Y = Ny = (AB^1–a)K = XK, where X equals AB^1–a. This puts the production function in notation used in the chapter.

        Investment is DK + dK = sY = national saving. Dividing through both sides of that expression by K and using the production function gives DK/K + d = sXK/K = sX, so DK/K = sX - d, which is the long-run growth rate of physical capital. Since output and human capital are proportional to physical capital, they will all grow at that same rate.