(b) and

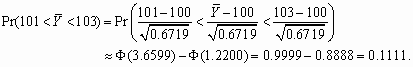
(c) and

(a)

(b) The standard error is SE

(c) The computed t-statistic is

Because of the large sample size we can use Equation (3.14) in the text to get the
p-value for the test vs.

(d) Using Equation (3.17) in the text, the p-value for the test vs. is

(e) Part (c) is a two-sided test and the p-value is the area in the tails of the standard normal distribution outside ± (calculated t-statistic). Part (d) is a one-sided test and the p-value is the area under the standard normal distribution to the right of the calculated t-statistic.

(f) For the test vs. we cannot reject the null hypothesis at the 5% significance level. The p-value 0.066 is larger than 0.05. Equivalently the calculated t-statistic is less than the critical value 1.645 for a one-sided test with a 5% significance level. The test suggests that the survey did not contain statistically significant evidence that the incumbent was ahead of the challenger at the time of the survey.

(a) 95% confidence interval for p is

(b) 99% confidence interval for p is

(c) The interval in (b) is wider because of a larger critical value due to a lower significance level.

(d) Since 0.50 lies inside the 95% confidence interval for p, we cannot reject the null hypothesis at a 5% significance level.

(a) The hypothesis test for the difference in mean monthly salaries is

The t-statistic for testing the null hypothesis is

Use Equation (3.14) in the text to get the p-value:

The extremely low level of p-value implies that the difference in the monthly salaries for men and women is statistically significant. We can reject the null hypothesis with a high degree of confidence.

(b) From part (a), there is overwhelming statistical evidence that mean earnings for men differ from mean earnings for women. To examine whether there is gender discrimination in the compensation policies, we take the following one-sided alternative test

With the t-statistic the p-value for the one-sided test is:

With the extremely small p-value, the null hypothesis can be rejected with a high degree of confidence. There is overwhelming statistical evidence that mean earnings for men are greater than mean earnings for women. However, by itself, this does not imply gender discrimination by the firm. Gender discrimination means that two workers, identical in every way but gender, are paid different wages. The data description suggests that some care has been taken to make sure that workers with similar jobs are being compared. But, it is also important to control for characteristics of the workers that may affect their productivity (education, years of experience, etc.). If these characteristics are systematically different between men and women, then they may be responsible for the difference in mean wages. (If this is true, it raises an interesting and important question of why women tend to have less education or less experience than men, but that is a question about something other than gender discrimination by this firm.) Since these characteristics are not controlled for in the statistical analysis, it is premature to reach a conclusion about gender discrimination.

1. (a)