Fraction Basics

Ratio

Lynn saved $400 and spent $500 of his last paycheck. The ratio of money saved to money spent is 4 to 5, that is, Lynn saved $4 for every $5 spent.

Ratio:  Ratios are used to compare quantities. The ratio of a to b can be written either in fraction notation as  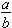or as a : b. 

The colon notation is specifically reserved for ratios. The fraction form makes sense in many contexts since in some cases a ratio may also be considered as a fraction. For example, in the above problem the ratio of money saved to the value of the paycheck is 4 to 9. We may also consider that four-ninths of the paycheck was saved. The ratio 4:9 conveys the same idea as the fraction 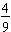in this context.

However, the concept of ratio is NOT interchangeable with the concept of fraction. In the above example, the ratio 4:5 does not make sense as a fraction because the amount saved is not a fractional part of the amount spent. Remember that the denominator of a fraction tells how many equal-sized pieces the whole is divided into. Therefore a fraction, by definition, ALWAYS compares to the whole (total). Ratios on the other hand may compare either to the whole or to another part. The above example illustrated each of these two types of ratios: the 4:5 would not make sense as a fraction; whereas, the ratio 4:9 conveys the same idea as a fraction.

Examples:  For instance, suppose a class of 30 students has 13 boys and 17 girls. 

The fraction of boys must be expressed as a part-to-whole expression. That is, it must compare the number of boys to the total of students (the whole class). The fraction of boys is 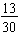.  And the ratio of boys to the class is 13:30. In this case, the problem may be thought of in terms of either a fraction or a ratio.

 

The ratio of the class to the boys is a whole-to-part expression. That is, it compares the whole class to the part of the class that is boys. The ratio of the class to boys is 30:13 and may be written as . But, in this case, it does NOT make sense to think of the whole class as being a fractional part of the boys.

 

The ratio of boys to girls is a part-to-part expression. That is, it compares the part of the class that is boys to the part of the class that is girls. The ratio of boys to girls is 13:17 and may also be written as  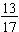. But, in this case, it does NOT make sense to think of the boys as being a fractional part of the girls.

.

Notice that the ratio of girls to boys is a different ratio. It is also a part-to-part expression, but it is 17:13 which may also be written as 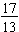. Again, it is does NOT make sense to think of the girls as being a fractional part of the boys.

 

 

 

return to top | previous page | next page