By now, you have probably recognized that some percents are equivalent fractions with which we are very familiar. When these percents occur with "compatible numbers" (numbers that simplify or multiply easily), we may calculate the percent problems mentally.
Example: 100% is the whole, so 100% of 782 is 782 since 1(782) = 782.
Example: 50% is one-half, so 50% of 84 is 42 since ½ (84) = 42.
Example: 25% is one-fourth, so 25% of 20 is 5 since ¼ (20) = 5.
Example: 10% is one-tenth (one place value less), so 10% of 36.5 is 3.65 since 0.1(36.5) = 3.65.
The shortcut is even more useful by realizing that due to the Commutative Property of Multiplication
, "28% of 50" must have the same final answer as "50% of 28".
This is due to the fact that the multiplication 
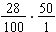must have the same answer as 
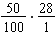since 
.
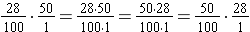So 100%, 50%, 25,% and 10% are not only easy percents to use when the numbers are compatible; they are also easy bases to take a percentage of, since 40% of 25 must have the same value as 25% of 40 (solution). These are not the same problem, but they have the same arithmetic answer. Since when we multiply the fraction forms, we can use the commutative property in the numerator and the arithmetic becomes identical.
Note: Here are some basic percent-fraction equivalences that should be memorized for use with mental estimation and compatible numbers.
Work these problems mentally.
Sandy has a credit card balance of $960. If the interest rate is 12½%, how much interest would Sandy owe?
Jamie has a $50,000 CD in Lake Region Bank. The APR (annual percentage rate) is 2.8%. How much interest will Jamie receive at the end of one year?
Three percent exceeds 2 percent by 50 percent, not by 1 percent.
Content ©2010. All Rights Reserved.
Date last modified: July 15, 2010.
Created with SoftChalk LessonBuilder
By Kris Montis and Timothy Peil
