5.3 Hilbert's Axioms Modified for Plane Elliptic Geometry
I have tried to avoid long numerical computations, thereby following Riemann's postulate that proofs should be given through ideas and not voluminous computations.
—David Hilbert (1862–1943)

Introductory Notes. 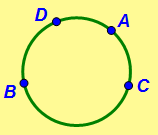Consider three distinct collinear points A, B, and C in the Riemann Sphere model. Depending on where we start, we could place the points in any of the following orders: A-B-C, A-C-B, B-A-C, B-C-A, C-A-B, and C-B-A. Hence, we cannot say one of the points is between the other two points. However, for four distinct collinear points A, B, C, and D, we could say that two of them separate the other two.  To cover this problem, we replace the Axioms of Order with the Axioms of Separation. For example, in the diagram on the right, point A and B separate points C and D.
     In order to use the Riemann Sphere model with the following axiom set, we identify each pair of antipodal points as a single point since two distinct lines are incident with a unique point, i.e., the Modified Riemann Sphere model.

Undefined Terms point, line, lie, separate, and congruence.

Group I. Axioms of Incidence

I.1.  For every two distinct points A and B, there exists a line m that contains each of the points A and B.
I.2.  For every two distinct points A and B, there is no more than one line m that contain each of the points A and B.
I.3.  There exist at least two distinct points on a line. There exist at least three points that do not lie on a line.
I.4.  (Elliptic Parallel Postulate) For any two distinct lines m and n, there exists a unique point A contained by lines m and n.

Group II. Axioms of Separation (or Axioms of Order)

II.1.  For any three distinct points A, B and C on a line m, there is a point D on m such that A and B separates C and D (denoted (A, B | C, D).
II.2.  If (A, B | C, D), then the points A, B, C, and D are distinct and collinear.
II.3.  If (A, B | C, D), then (B, A | C, D) and (C, D | A, B).
II.4.  If (A, B | C, D), then A and C does not separate B and D.
II.5.  For any four distinct collinear points A, B, C, and D, (A, B | C, D) or (A, C | B, D) or (A, D | B, C).
II.6.  For any five distinct collinear points A, B, C, D, and E, if (A, B | D, E), then either (A, B | C, D) or (A, B | C, E).
II.7.  Separation of points is invariant under a perspectivity, i.e., if (A, B | C, D) and there is a perspectivity mapping A, B, C, and D on line p to the corresponding points A', B', C', and D' on line p', then (A', B' | C', D').

Since in neutral geometry the definitions of segments, rays, angles, and triangles all depended on betweenness of points, the definitions all need to be revised based on the separation of points.

Exercise 5.1.1. Use the concepts from the separation axioms to write definitions for each of the following.

(a)  segment    
(b)  ray   (Note that in the spherical model a ray is a line.)
(c)  angle  (Note that at the vertex of an angle there are four angles. Consider how to distinguish which is the particular angle desired.)
(d)  triangle  (Note that three noncollinear points determine more than one triangle.)

Group III. Axioms of Congruence

III.1.  If A, B are two points on a line m, and A' is a point on the same or on another line m' then it is always possible to find a point B' on a given side of the line m' through A' such that the segment AB is congruent to the segment A'B' (denoted AB ≅ A'B').
III.2.  Congruence of segments is an equivalence relation.
III.3.  If two segments are congruent, then their mutually complementary segments are congruent.
III.4.  On the line m let AB and BC be two segments which except for B have no point in common. Furthermore, on the same or on another line m' let A'B' and B'C' be two segments which except for B' also have no point in common. In that case, if AB ≅ A'B' and BC ≅ B'C', then AC ≅ A'C'.
III.5.  Let ∠(h,k) be an angle and m' a line and let a definite side of m'. Let h' be a ray on the line m' that emanates from the point O'. Then there exists one and only one ray k' such that the angle ∠(h,k) is congruent to the angle ∠(h',k') and at the same time all interior points of the angle ∠(h',k') lie on the given side of m'. Symbolically ∠(h, k) ≅  ∠(h',k').  Every angle is congruent to itself.
III.6.  If for two triangles ABC and A'B'C' the congruences AB ≅ A'B', AC ≅ A'C', ∠BAC ≅ ∠B'A'C' hold, then the congruence ∠ABC ≅ ∠A'B'C'  is also satisfied.

Group IV. Axiom of Parallels - Elliptic geometry has no parallel lines and hence the elliptic parallel postulate should probably be called the elliptic no parallels postulate.

See Group I. Axioms of Incidence - I.4.  (Elliptic Parallel Postulate) For any two distinct lines m and n, there exists a unique point A contained by lines m and n.

Group V. Axiom of Continuity

V.  (Dedekind's Axiom)  Assume the set of all points on a segment AB is the union, S₁ U S₂, of two nonempty subsets of segment AB such that no point of S₁ separates two points of S₂. Then there is a unique point C in the interior of segment AB such that (A, C | P₁, P₂) if and only if P₁ is in S₁ and P₂ is in S₂ and C is neither P₁ nor P₂.

Defined Terms

• Consider two distinct points A and B on a line m, then there are points C and D on line m where (A, B | C, D). The union of the set of the two points A and B and the set of all points X where (A, B | C, X) is called segment AB/C. The points A and B are called the endpoints of the segment AB/C. The set of all points X where (A, B | C, X) is called interior of segment AB/C. The set of all points Y where (A, B | Y, D) is called exterior of segment AB/C. The segment AB/C and segment AB/D are called mutually complementary segments.  Note that three points are often needed to name a segment since points A and B define two mutually complementary segments, segment AB/C and segment AB/D. Further note that the interior of segment AB/C is the exterior of segment AB/D.
• Let A, A', O, B be four points of a line m such that O and B do not separate A and A'. The points A, A' are then said to lie on the line m on one and the same side of the point O and the points A, B are said to lie on the line m on different sides of the point O. The totality of the points of the line m that lie on the same side of O is called a ray emanating from O. The point O is the vertex of the ray emanating from O and A is a relative point of the ray emanating from O.
• Let h and k be any two distinct rays emanating from O and lying on distinct lines. Further, let AB  be a segment with A and B relative points of rays h and k, respectively, distinct from O.  The pair of rays h, k is called an angle and is denoted by ∠(h,k) or ∠(k,h) or ∠AOB. Let C be an exterior point of segment AB. All points that lie in the interior of segments XY/Z where X and Y are relative points of h and k, respectively, and Z is a point on line OC  are said to lie in the  interior of the angle ∠(h,k) or ∠AOB.
• Let AB, BC, and CA be three segments where A, B, and C are three points which do not lie on the same line. The union of the three segments AB, BC, and CA is a triangle called triangle ABC. (Note that the noncollinear points A, B, and C define more than one triangle.)

Exercise 5.1.2. (a) Prove a segment has at least three points.
                         (b) Prove a segment has infinitely many points.

Exercise 5.1.3.  Show that the Riemann Sphere where each pair of antipodal points is a point satisfies each of the axioms.

_____________
Coxeter, H. S. M., Non-Euclidean Geometry, Fourth Edition. University of Toronto Press, 1961.
Greenberg, Marvin J., Euclidean and Non-Euclidean Geometries: Development and History. San Francisco: W. H. Freeman and Company, 1974.
Hilbert, David, Foundations of Geometry (Grundlagen der Geometrie), Second English Edition trans. by Unger,L. LaSalle: Open Court Publishing Company, 1971 (1899).
Riemann geometry. L.A. Sidorov (originator), Encyclopedia of Mathematics. URL: http://www.encyclopediaofmath.org/index.php?title=Riemann_geometry&oldid=15725