Readings for Session 8 – Addition and Subtraction of Whole Numbers
Addition of Whole Numbers: Let a = n(A) and b = n(B) where A and B are two disjoint finite sets. Then a + b = n(A ∪ B).
|
|
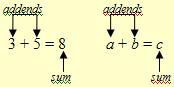 |
Properties for Addition
of Whole Numbers
Commutative Property of Addition:
The
Commutative Property for Addition of Whole Numbers
says that the
order of the addends does not change the sum.
General Property:
a +
b =
b +
a
Relationship to sets: Use the set definition for addition and the Commutative Property for the Union of Sets.
Let
A and
B be two disjoint
finite sets with cardinal numbers
n(A)
and n(B).
Then
n(A)
+ n(B)
= n(A
∪
B) =
n(B
∪
A) =
n(B)
+ n(A).
Numeric Example:
3 + 5 = 8 = 5 + 3
Algebraic Example:
3x + 4x
= 7x = 4x
+ 3x
Associative Property of
Addition:
The
Associative Property for
Addition of Whole Numbers says that how the addends are
grouped does not change the sum.
General Property:
(a +
b) +
c =
a + (b
+ c)
Relationship to sets: Use the set definition for addition and the Associative Property for the Union of Sets.
Let
A,
B, and
C be three disjoint
finite sets with cardinal numbers
n(A),
n(B),
and n(C).
Then
[n(A)
+ n(B)]
+ n(C)
= n([A
∪
B]
∪
C)
= n(A
∪ [B
∪
C])
= n(A)
+ [n(B)
+ n(C)].
Numeric Example:
(2 + 5) + 8 =
7 + 8
= 15
= 2 + 13
= 2 + (5 + 8)
Algebraic Example:
(2x + 3x)
+ 4x
= 5x + 4x
= 9x
= 2x + 7x
= 2x + (3x
+ 4x)
Identity Property of Addition:
The Identity Property for
Addition of Whole Numbers says that the sum of a number and
zero is the number. Zero is called the
additive identity.
General Property:
a + 0 = 0 +
a =
a
Relationship to sets: Use the set definition for addition and the Identity Property for the Union of Sets.
Let A be a finite set
with cardinal number n(A).
Then
n(A)
+ n(∅)
= n(A
∪
∅)
= n(A).
Numeric Example:
5 + 0 = 5
and 0
+ 5 = 5
Algebraic Example: 3x + 0 = 3x and 0 + 3x = 3x
![]()
Return
to Peil's Homepage | Minnesota
State University Moorhead | Mathematics
Department