Readings for Session 8 – (Continued)
Solving Equations with Properties of Equality
Solution to an Equation:
A value is a solution to an equation when that value is substituted for
the variable in the equation, the resulting arithmetic statement
is a true statement.
Since 4 + 3 = 7, it is
true that 3 is a solution to the equation.
We state that 3 is a solution to this equation by writing
x = 3.
We write the solution set to the equation as {3}.
Example:
The value 4 is not
a solution to the equation 10 –
x = 5 since 10 – 4 ≠
5.
Example: The value 30 is a solution to x – 12 = 18 since 30 – 12 = 18.
The solution set for the
equation is {30}.
Addition Property of Equality: If we add the same amount
to both sides of an equation, the resulting statement is still
an equation and it has the same solution set as the original
equation.
General Property:
If
a =
b, then
a + c = b +
c.
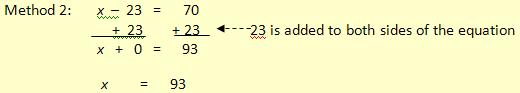
Example:
Solve x – 23 =
70. We illustrate two methods.
Method 1:
x – 23 = 70
(x
– 23) + 23 = 70 + 23
Addition Property of Equality
x = 93
Check the solution by substitution:
93 – 23 = 70.
Subtraction Property of Equality: If we subtract the same
amount from both sides of an equation, the resulting statement
is still an equation and it has the same solution set as the
original equation.
General Property:
If
a =
b, then
a – c = b –
c.
Example:
Solve x + 19 =
59. We illustrate two methods.
Method 1:
x + 19
= 59
(x
+ 19) – 19 = 59 – 19
Subtraction Property of Equality
x = 40
Check the solution by substitution: 40 + 19 = 59.
![]()
Return
to Peil's Homepage | Minnesota
State University Moorhead | Mathematics
Department