Exercise 2.1. All triangles are isosceles triangles.
![]() Printout
Printout
The truth of a theory is in your
mind, not in your eyes.
—![]() Albert Einstein (1879–1955)
Albert Einstein (1879–1955)
Statement. All
triangles are isosceles triangles.
(a) Identify the error/s in the
proof. Is the error a logic error? A bad assumption? Explain.
(b) Identify and write a definition for each term used in the statement of the
theorem and proof.
(c) Identify and state any assumptions made in the proof.
(d) Identify and state any theorems used in the proof.
(Hint. If you are unable to find the
errors from reading the proof, use dynamic geometry software - such as
Geometer's Sketchpad or
GeoGebra - to construct the
figure based on the steps in the proof.)
Proof. Given any Let ray r
be the bisector of
Let M
be the midpoint of segment AB. Let l be the line perpendicular to segment AB at M. Let D be the point of intersection of ray r and line l.
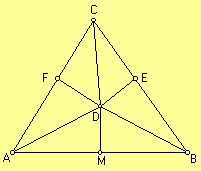
Case 1.Assume D = M. We have and
Then
,
since line l is perpendicular to
segment AB at M. Since M is the
midpoint of segment AB,
Also,
Hence, by SAS,
Thus,
and
is an isosceles triangle.
Case 2. Assume D is distinct from M. Then
,
since line l is perpendicular to
segment AB at M. Since M is the
midpoint of segment AB,
Also,
Hence, by SAS,
Thus
Let E
be the foot of the perpendicular line from D
to line BC. Let F be the foot of the perpendicular line from D to line AC. Then
and
are right angles. Thus
Since ray r
bisects
,
Also,
Hence, by AAS,
Thus
and
Since
,
,
and
and
are right angles, by HL,
Hence,
Since
and
,
we have that
AC = AF + FC = BE + EC = BC.
Hence, and
is an isosceles triangle.
Therefore, since the triangle was
arbitrarily chosen, all triangles are isosceles.//