TOC & Ch. 0 & Ch. 1 Axiom Table of Contents Ch. 0 Introduction Ch. 1 Axiomatic Systems 1.1.1 Introduction 1.1.2 Examples 1.1.3 History 1.2 A Finite Geometry 1.3 Finite Projective 1.4 Applications

Ch. 2 Neutral Geometry Ch. 2 Table of Contents 2.1.1 Introduction 2.1.2 History 2.1.3 Analytic Models 2.2 Incidence Axioms 2.3 Distance/Ruler Axioms 2.4.1 Plane Separation Axiom 2.4.2 Angle & Measure 2.5.1 Supplement Postulate 2.5.2 SAS Postulate 2.6.1 Parallel Lines 2.6.2 Saccheri Quadrilateral 2.7.1 Euclid Parallel Postulate 2.7.2 Hyperbolic Parallel Postulate 2.7.3 Elliptic Parallel Postulate 2.8 Euclid/Hyperbolic/Elliptic Birkhoff's Axioms Hilbert's Axioms SMSG Axioms

Ch. 3 Transformational Ch. 3 Table of Contents 3.1.1 Introduction 3.1.2 History 3.2.1 Definitions 3.2.2 Analytic Model 3.2.3 Affine Transformation 3.3.1 Isometry 3.3.2 Model/Collinearity 3.3.3 Model/Isometry 3.4.1 Direct Isometry 3.4.2 Model/Direct 3.5.1 Indirect Isometry 3.5.2 Model/Indirect 3.6.1 Similarity Transformation 3.6.2 Model/Similarity 3.7 Other Affine Transformations

Ch. 4 Projective Geometry Ch. 4 Table of Contents 4.1.1 Introduction 4.1.2 Historical 4.2.1 Axioms 4.2.2 Basic Theorems 4.3 Duality 4.4 Desargue's Theorem 4.5.1 Harmonic Sets 4.5.2 Music & Harmonic Sets 4.6.1 Definitions for Projectivity 4.6.2 Fundamental Theorem 4.6.3 Projectivity/Harmonic Sets 4.6.4 Alternate Construction 4.7.1 Conics 4.7.2 Pascal's Theorem 4.7.3 Tangents to Conics

Other Topics Ch. 5 Spherical Geometry Ch. 6 Fractal Geometry Ch. 7 Topology

Appendices Internet Resources Index Geometer's Sketchpad/GeoGebra JavaSketchpad/GeoGebraHTML Video Lectures Logic Review References Acknowledgements

        2.4.1 Plane Separation Postulate  Printout
Whoever… proves his point and demonstrates the prime truth geometrically should be believed by all the world, for there we are captured.
—Albrecht Dürer (1471–1528)

 

      An important axiom that is often not considered in a high school geometry course is the Plane Separation Axiom. The Missing Strip plane is a model that satisfies all the axioms we have discussed to this point. It is included for the sole purpose of demonstrating the necessity for the Plane Separation Axiom.

Definition. A set S is convex if for every two points P and Q in S, the segment  is a subset of S.

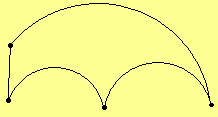
      Consider the interiors of the above three figures. Note that the first and last figures have segments that are not completely contained in their interiors. The first and last sets are not convex. It is important to note that the definition of convex depends on a segment; a set that is convex in the Poincaré Half-plane may not be convex as a set in the Euclidean plane. Consider the interior of the following quadrilateral constructed in the Poincaré Half-plane. The set is convex in the Poincaré Half-plane but is not a convex set in the Euclidean plane.

Postulate 9. (Plane Separation Postulate) Given a line and a plane containing it, the points of the plane that do not lie on the line form two sets such that: (i)  each of the sets is convex; and (ii)  if P is in one set and Q is in the other, then segment  intersects the line.

Definitions. Each of the two convex sets is called a half-plane, and the line is called the edge. Points in the same half-plane are said to be on the same side. Two points in different half-planes determined by a line are said to be on opposite sides.

      Note that the line and the two half-planes are disjoint sets; that is, a line forms three disjoint sets, the line and two half-planes. To illustrate the Plane Separation Postulate, consider the Cartesian plane, Missing Strip plane, and Poincaré Half-plane.

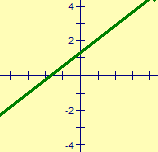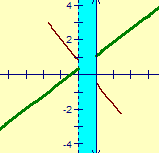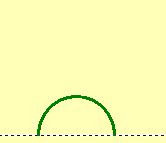      The line in the Cartesian plane separates the plane into two convex sets, the region above the line and the region below the line. The line in the Missing Strip plane does not separate the plane into two convex sets. See the illustration, the segment shown does not intersect the line since the light blue region between 0 and 1 does not exist in the plane. The line in the Poincaré Half-plane separates the plane into two convex sets. (Remember the shape of a line and segment in the Poincaré Half-plane.)
      A statement that is sometimes used in place of the Plane Separation Postulate is Pasch's Postulate. It can be proven that the Plane Separation Postulate and Pasch's Postulate are equivalent, Moritz Pasch (1843-1930). Since this is a survey course, we will not prove their equivalency here. (The proofs may be found in books on the foundations of geometry such as Geometry: A Metric Approach with Models by Millman and Parker.)

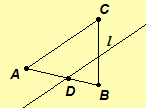
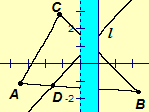
Theorem 2.6 (Pasch's Postulate). For any line l, any triangle , and any point D on l such that D is between A and B, then either l intersects segment AC or l intersects segment BC.

      The above diagrams illustrate one example of Pasch's Postulate in the Euclidean plane, Missing Strip plane, and Poincaré Half-plane. Is Pasch's Postulate satisfied in each plane?

      Reminder. Prepared Geometer's Sketchpad sketches and GeoGebra sketches with tools for constructions in the Missing Strip plane and Poincaré Half-plane are available in Appendix B of the Course Title Page - Geometer's Sketchpad and GeoGebra Prepared Sketches and Scripts. Also, an on-line java based program called NonEuclid may be used for constructions in the Poincaré Half-plane at http://cs.unm.edu/~joel/NonEuclid/NonEuclid.html.

Exercise 2.28. Is the Plane Separation Postulate discussed or mentioned in the high school geometry book you are using as a reference?

Exercise 2.29. Is the Plane Separation Postulate independent of the other axioms? Explain.

Exercise 2.30. Find an analytic example that shows the Missing Strip plane does not satisfy Pasch's Postulate.

Exercise 2.31. Prove Pasch's Postulate.