TOC & Ch. 0 & Ch. 1 Axiom Table of Contents Ch. 0 Introduction Ch. 1 Axiomatic Systems 1.1.1 Introduction 1.1.2 Examples 1.1.3 History 1.2 A Finite Geometry 1.3 Finite Projective 1.4 Applications

Ch. 2 Neutral Geometry Ch. 2 Table of Contents 2.1.1 Introduction 2.1.2 History 2.1.3 Analytic Models 2.2 Incidence Axioms 2.3 Distance/Ruler Axioms 2.4.1 Plane Separation Axiom 2.4.2 Angle & Measure 2.5.1 Supplement Postulate 2.5.2 SAS Postulate 2.6.1 Parallel Lines 2.6.2 Saccheri Quadrilateral 2.7.1 Euclid Parallel Postulate 2.7.2 Hyperbolic Parallel Postulate 2.7.3 Elliptic Parallel Postulate 2.8 Euclid/Hyperbolic/Elliptic Birkhoff's Axioms Hilbert's Axioms SMSG Axioms

Ch. 3 Transformational Ch. 3 Table of Contents 3.1.1 Introduction 3.1.2 History 3.2.1 Definitions 3.2.2 Analytic Model 3.2.3 Affine Transformation 3.3.1 Isometry 3.3.2 Model/Collinearity 3.3.3 Model/Isometry 3.4.1 Direct Isometry 3.4.2 Model/Direct 3.5.1 Indirect Isometry 3.5.2 Model/Indirect 3.6.1 Similarity Transformation 3.6.2 Model/Similarity 3.7 Other Affine Transformations

Ch. 4 Projective Geometry Ch. 4 Table of Contents 4.1.1 Introduction 4.1.2 Historical 4.2.1 Axioms 4.2.2 Basic Theorems 4.3 Duality 4.4 Desargue's Theorem 4.5.1 Harmonic Sets 4.5.2 Music & Harmonic Sets 4.6.1 Definitions for Projectivity 4.6.2 Fundamental Theorem 4.6.3 Projectivity/Harmonic Sets 4.6.4 Alternate Construction 4.7.1 Conics 4.7.2 Pascal's Theorem 4.7.3 Tangents to Conics

Other Topics Ch. 5 Spherical Geometry Ch. 6 Fractal Geometry Ch. 7 Topology

Appendices Internet Resources Index Geometer's Sketchpad/GeoGebra JavaSketchpad/GeoGebraHTML Video Lectures Logic Review References Acknowledgements

           3.3.3 Isometry for the Analytic Euclidean Plane Model Printout
In mathematics the art of asking questions is more valuable than solving problems.
—Georg Cantor (1845–1918)

       A natural question arises, "What is the form of the matrix of an isometry that is an affine transformation of the Euclidean plane?"  We investigate that question. The matrix of an affine transformation of the Euclidean plane has the form . What restrictions need to be placed on the values a_ij for A to be a matrix of an isometry? For two points X and Y, let X' = AX and Y' = AY. Then

 and  

If we assume A is an isometry, then d(X, Y) = d(X', Y'). Hence

 

For the first and last expressions to be equal, we must have

 

      Assume a₁₁ = 0. Then by (1), a₂₁ = ±1. And by (3), a₂₂ = 0. Finally, by (2), a₁₂ = ±1.
      Assume a₁₁ is nonzero. Then by (3),  Substitute into (2),  Hence, by (1), a₂₂ = ± a₁₁. If a₂₂ = a₁₁, then (3) implies a₁₂ = –a₂₁. If a₂₂ = –a₁₁, then (3) implies a₁₂ = a₂₁.

      Since , there is a real number  such that  and Further, note that there are no restrictions on a₁₃ and a₂₃.
      Thus, we summarize the results in the following proposition, which only has the converse left to be proven.

Proposition 3.7. An affine transformation of the Euclidean plane is an isometry if and only if the matrix representation is

 (direct isometry) or  (indirect isometry).

 Corollary to Proposition 3.7. The determinant of a direct isometry is 1 and the determinant of an indirect isometry is –1.

 

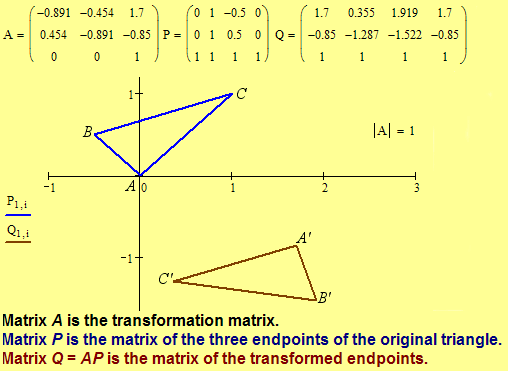
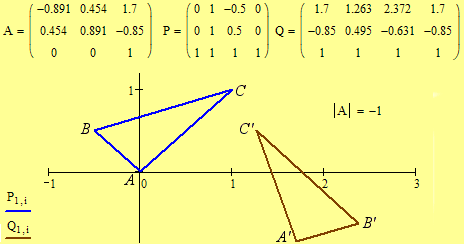
Examples. Which is a direct isometry? Which is an indirect isometry? Note the positions of the triangles. What happens with the measures of the angles between the sides? Use the definition of the measure of the angle between two lines to check your conjectures. Investigate further by looking at the animation video clips. (See below between the two examples for the links.)

Click here for an animation of the graphic examples: example above or example below.

Proposition 3.8. The product of the matrices of two affine direct or two affine indirect isometries of the Euclidean plane is the matrix of an affine direct isometry. Further, the product of an affine direct and an affine indirect isometry of the Euclidean plane is an affine indirect isometry of the Euclidean plane.

Proposition 3.9. The set of affine direct isometries of the Euclidean plane is a group.

Proposition 3.10. The set of affine isometries of the Euclidean plane is a group.

      We partially examine the questions asked before the illustrations above. Notice that the first diagram illustrates a direct isometry; whereas, the second diagram is an indirect isometry. If we label the vertices in the original triangle clockwise as A, B, and C, what happens with the vertices in the image figure for each diagram? In the first diagram, the vertices of the image remain in the same clockwise order but reverse to a counter-clockwise order in the second diagram. It appears that a direct isometry keeps the orientation the same, and an indirect isometry reverses the orientation.
      Examine this further by computing the measures of the angles between the lines determined by the sides for both diagrams. The angle  between the lines l[1, –1, 0] and m[1, –3, 2] measures approximately –0.464, where  (Check the computations determining the two lines and the measure of the angle.) The measure of the angle  between the two image lines l'[1, –3.085, –4.322] and m'[1, 6.655, 8.210], in the first diagram, is approximately –0.464. The angles between the lines l and m and the lines l' and m' measure the same. The measure of the angle  between the two image lines l'[1, 0.325, –1.424] and m'[1, 0.985, –1.751], in the second diagram, is approximately 0.464. The measure of the angle between the image lines l' and m' has the opposite sign of the measure of the angle between the lines l and m. Compute the values for the other two angles,  and .
      The observations in the above examples lead us to conjecture the next two propositions.     

Proposition 3.11. For an affine direct isometry of the Euclidean plane, the measure of the angle between two lines equals the measure of the angle between the two image lines.

Proof. Let the lines p' and q' be the images of lines p and q under a direct isometry with matrix A.  Let B be the inverse of the matrix A. By Proposition 3.9, B is the matrix of a direct isometry. By Proposition 3.6, there are nonzero real numbers k₁ and k₂ such that k₁p' = pB and k₂q' = qB. We use the results of the previous two sentences together with Proposition 3.7 to compute .  The line q' may be expressed in a similar form. Compute the measure of the angle between p' and q' where the angle is not a right angle. (The case for a right angle is left for you to verify.)

 

 

Proposition 3.12. For an affine indirect isometry of the Euclidean plane, the measure of the angle between the two image lines has the opposite sign of the measure of the angle between the two lines.

Exercise 3.37. Let A(0, 0, 1), B(1, 0, 1), C(0, 1, 1), D(1, 1, 1), E(2, 1, 1), and F(1, 2, 1). Show the sets {A, B, C} and {D, E, F} are congruent. (Two sets of points are said to be congruent provided there is an isometry where one set is the image of the other set.)

Exercise 3.38. An affine transformation maps X(5, 0, 1) to X'(4, 6, 1) and Y(0, 0, 1) to Y'(1, 2, 1).  (a)  Show d(X, Y) = d(X', Y') and show the transformation may not be an isometry.  (b)  Find a direct isometry for the transformation.  (c)  Find an indirect isometry for the transformation.  (d)  Find the image of Z(3, 10, 1) for the isometries you obtained in parts (b) and (c).

Exercise 3.39. Complete the proof of Proposition 3.7.

Exercise 3.40. Prove Proposition 3.8.

Exercise 3.41. Prove Proposition 3.9.

Exercise 3.42. Prove Proposition 3.10.

Exercise 3.43. Fill in the missing steps of the two computations in the proof of Proposition 3.11.

Exercise 3.44. Prove the inverse of an affine indirect isometry of the Euclidean plane  is an affine indirect isometry of the Euclidean plane.

Exercise 3.45. Prove Proposition 3.12. (Note Exercise 3.44.)