Prove Theorem 4.1.
The more we do, the more we can do.
—
Solution Basic Theorems
Exercise 4.1.
Prove Theorem 4.1.
The more we do, the more we can do.
—![]() William
Hazlitt (1778–1830)
William
Hazlitt (1778–1830)
Important Note. You must begin the proof by assuming two distinct lines, even though we have not shown the existence of any lines. But this is not a problem, since the statement is valid if no lines or only one line exist. The statement of the theorem is equivalent to "If two distinct lines exist, then they are incident with exactly one point." Remember from logic that a conditional is always true when the antecedent is false.
Theorem 4.1. (Dual of Axiom 1) Any two distinct lines are incident with exactly one point.
Proof. Let l1 and l2 be two distinct lines. By Axiom 2, there is at least one point P where lines l1 and l2 are incident with it. Suppose there is a second point Q with lines l1 and l2 incident with it. Then the two points P and Q would be incident with line l1 and with line l2. But this contradicts Axiom 1, since two distinct points are incident with exactly one line. Therefore, any two distinct lines are incident with exactly one point. //
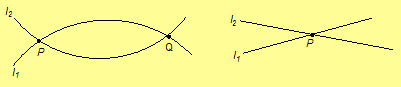
|
© Copyright 2005, 2006 - Timothy Peil |