Decimal Fractions

Terminating Decimals and Repeating Decimals

Convert Decimal Fractions to Common Fraction

Terminating Decimals

Decimals that are not repeating decimals are called terminating decimals. Terminating decimals represent fractions that can be expressed with a denominator that is a power of 10. To change a terminating decimal to an equivalent common fraction, all we need to do is use place value to determine the denominator. Suggestion: Verbalize the decimal by reading it correctly, that is, read 0.025 as twenty-five thousandths.

Example:

Self-Check Problems

Convert 0.35 and 0.175 to a simplified common fractions.

Solution for 0.35 --------------- Solution for 0.175

Repeating Decimals

Repeating decimals can be changed to common fractions using an algebraic method. The method is to multiply the number by a power of ten that shifts the decimal point the number of place values that are repeating, and then subtract the number from that new value. This will cancel out the repeating portion.

Example: We change 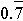to a common fraction.

First we notice that multiplying by 10 moves the value one place value to the left in the place value table. For example, 7(10) =70 and this places the digit 7 one place value to the left of where it was in the original value of 7. Or, 0.77(10) = 7.7.

Let 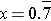, then   (just moving all the digits one place value to the left).
We then subtract these two values, and the repeating parts subtract completely off.

We solve this equation for x to obtain .

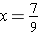Example: We change to a common fraction.

First we notice that multiplying by 100 moves the two place values to the right, i.e., .

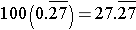Let 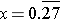, then 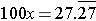  (just move all digits two place values to the left). We then subtract these two values, and the repeating parts subtract completely off.

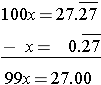
We then solve this equation for x and simplify to obtain .

Example: We change 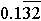to a common fraction.

Since there are only two positions repeating (the 32), we multiply by 100.

Let 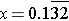, then 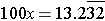. The digits were all moved two place values to the left and the repeating part is still the 32.

After solving the equation and simplifying, we obtain .

Self-Check Problems

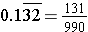Convert each repeating decimal to a simplified common fraction.

	Solution
	Solution

 

Joke or Quote

The polite mathematican says, "You go to infinity — and don't hurry back."

return to top | previous page