Methods of Simplifying Multiplication
Two Methods of Simplifying
One method is to use prime factorization and then divide out the common factors between the numerator and denominator.
Example:
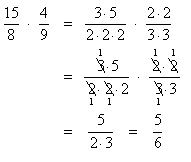
A second method is to divide out the common factors without completing the prime factorization of each term.
Example:
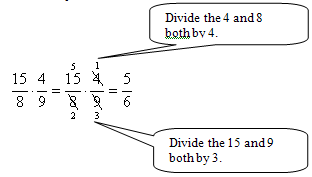


Two Final Reminders about Simplifying by Dividing Common Factors
- We may only simplify by dividing common factors when multiplying fractions. We cannot simplify by dividing common factors when adding or subtracting fractions. We use basic examples with models to help understand this rule.
'
Example:
Note here when we are multiplying, we may simplify by dividing common factors.

Note here when we are adding, we cannot simplify by dividing common factors.

- We must always use one factor in the numerator and one factor in the denominator when dividing common factors because it is the division bar (fraction bar) that we are interpreting as divided by.
return to top | previous page | next page
