Inspiration is needed in geometry, just as much as in poetry.
—
1.3 A Finite Projective Plane Geometry
![]() Printout
Printout
Inspiration is needed in geometry, just as much
as in poetry.
—![]() Aleksandr Sergeyevich Pushkin (1799–1837)
Aleksandr Sergeyevich Pushkin (1799–1837)
![]() Axioms for a Finite Projective Plane
Axioms for a Finite Projective Plane
Undefined Terms. point, line, and incident
Defined Term. Points incident to the same line are collinear.
Axiom P1. For any two distinct points, there is exactly one line incident with both points.
Axiom P2. For any two distinct lines, there is at least one point incident with both lines.
Axiom P3. Every line has at least three points incident with it.
Axiom P4. There exist at least four distinct points of which no three are collinear.
The axiom system does not specify
the number of points on a line. As will be seen with the following examples,
finite models with different numbers of points can be constructed. Hence, we
define these different
![]() finite projective planes.
finite projective planes.
Definition. A projective plane of order n is a geometry that satisfies the above axioms for a finite projective plane and has at least one line with exactly n + 1 (n > 1) distinct points incident with it.
Theorem P1. There exists a projective plane of order n for some positive integer n.
The proof of the above theorem follows immediately from Axiom P3 and the following models since Axiom P3 guarantees the existence of a projective plane of order 2. Hence, we say that a projective plane of order n is well-defined.
First, we consider a projective
plane of order 2. Note that the models used for Fano's Geometry satisfy these axioms for a
projective plane of order 2. The reader should verify the models satisfy the
axioms to show that
this is in fact true.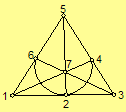
|
points |
lines |
|
A, B, C, D, E, F, G |
ADB, AGE, AFC, BEC, BGF, CGD, FDE |
Next, we give a model of a projective plane of order 3. In this case, there exists at least one line with exactly 4 distinct points incident with it.
|
points |
lines |
|
A, B, C, D, E, F, G, H, I, J, K, L, M |
ABCD, AEFG, AHIJ, AKLM, BEHK, BFIL, BGJM, CEIM, CFJK, CGHL, DEJL, DFHM, DGIK |
The reader should verify at least some of the cases.
Several questions or conjectures arise from these
two models. First, the above examples seem to indicate that the number of points
and lines in a projective plane of order n are the same. Second, every
point has the same number of lines incident to it, as well as, every line has
the same number of points incident to it. Third, how many points or lines are in
a projective plane of order n? Later in this section, we will state these
conjectures as theorems and prove them. (See Theorems P2-P5 below.)
Another natural question arises from these
two models: For what values of n does a projective plane of order n
exist? What is mathematically interesting is that this question has not been
completely answered. The question is an unsolved problem in mathematics, though
partial results have been obtained. In 1906, in a paper published in the
Transactions of the American Mathematical Society, Oswald Veblen and W. Bussey
proved that there exist finite projective planes of order pm
where p
is a prime number and m is a positive integer. Hence, there are projective planes of orders 4, 5,
7, 8, 9, 11, 13, 16, 17, 19, 23, 25, 27, etc. The conjecture is that the only
orders for which there is a projective plane of order n is when n is
a prime number to some positive integer power. A result by Bruck and Ryser in 1949 partially proved the
conjecture. Their result stated that there is no projective plane of order n,
if n is congruent to 1(mod 4) or 2(mod 4), and n cannot be written
as the sum of two squares. This result showed that n cannot be 6, 14, 21,
22, etc. Currently, the lowest order for which the conjecture has not been
proven is 12.
Definition. Lines incident to the same point are concurrent.
Exercise 1.12. How many cases for each axiom need to be considered to verify the model for a projective plane of order 3?
Exercise 1.13. Write the dual for the axioms of a finite projective plane.
Exercise 1.14. Prove the Dual of Axiom P1.
Exercise 1.15. Prove the Dual of Axiom P2.
Exercise 1.16. Prove the Dual of Axiom P3.
Exercise 1.17. Prove the Dual of Axiom P4.
![]() With the completion of the proof
for Exercises 1.14–1.17, you have shown that a finite projective
geometry satisfies the
principle of duality. Thus, when a theorem has been proven, a theorem for
the dual follows without writing a new proof, i.e. "Two for the price of one."
With the completion of the proof
for Exercises 1.14–1.17, you have shown that a finite projective
geometry satisfies the
principle of duality. Thus, when a theorem has been proven, a theorem for
the dual follows without writing a new proof, i.e. "Two for the price of one."
Theorem P2. In a projective plane of order n,
there exists at least one point with exactly n + 1 distinct lines
incident with it.
Proof. By the definition of a projective plane of order n,
there exists a line l with exactly n + 1 points incident to it,
call them P1, P2,
...
,Pn+1. By Axiom P4, there is a point Q not
incident with l. Thus by Axiom P1, there exist lines QP1,
QP2, ... ,QPn+1.
We need to show the lines are distinct and that there are no other lines
through Q.
Suppose QPi
= QPj for some i
≠ j.
Then by Axiom P1, Q, Pi, and Pj
would be on line l = PiPj , but this
contradicts that Q is not on line l. Hence, the n + 1 lines
QP1, QP2,
...
,QPn+1 are distinct.
Now let m be a line incident
to Q. By Axiom P2, lines l and m are incident with a point
R. Since P1, P2,
... ,Pn+1 are the only points
incident to l, R = Pi for some i
ϵ
{1,
... ,n + 1}. Hence, m = QR = QPi
which is one of the n + 1 lines through Q. Therefore, the
point Q is incident with exactly n + 1 lines.//
Since the
finite projective plane satisfies the principle of duality, the proof of Theorem
P2 could have been much shorter.
![]() Theorem P2 is the dual of the definition of a
projective plane of order n. Hence, the Theorem P2 follows immediately
from the principle of duality. The first proof was given to illustrate a proof
form that may be useful when proving other theorems.
Theorem P2 is the dual of the definition of a
projective plane of order n. Hence, the Theorem P2 follows immediately
from the principle of duality. The first proof was given to illustrate a proof
form that may be useful when proving other theorems.
Theorem P3. In a projective plane of order n, every point is incident with exactly n + 1 lines.
Proof. Let P be a point in a projective plane of order n. By the definition of a projective plane of order n,
there exists a line l with exactly n + 1 points incident to it,
call them P1, P2,
...
,Pn+1. Now either P is incident to l
or P is not incident to l.
Case 1. Assume P is not on
line l. The proof of this case is the exact same as the proof of Theorem
P2 with Q of Theorem P2 replaced with P. Therefore, P is
incident with exactly n + 1 lines.
Case 2. Assume P is on line
l. To prove this case, we show that there exists a line m such that
P is not incident with m and m has n + 1 distinct
points, then apply Case 1.
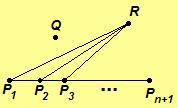 First, note P is one of the points P1, P2,
...
,Pn+1. Hence P = Pi for
some i
ϵ
{1,
... ,n +1}. By Axiom P4, there are distinct points Q
and R not
incident with l. By Axiom P1 and Axiom P3, lines RP1,
RP2, and RP3 exist. Further, since R
is not on l, by Axiom P1, P cannot be on at least two of the three
lines RP1, RP2, and RP3.
Similarly, Q cannot be on at least two of the three lines RP1,
RP2, and RP3. Hence, at least one
of the lines RP1, RP2, and RP3
has neither P nor Q incident with it. Therefore, there is
a line m with neither P nor Q incident with it.
First, note P is one of the points P1, P2,
...
,Pn+1. Hence P = Pi for
some i
ϵ
{1,
... ,n +1}. By Axiom P4, there are distinct points Q
and R not
incident with l. By Axiom P1 and Axiom P3, lines RP1,
RP2, and RP3 exist. Further, since R
is not on l, by Axiom P1, P cannot be on at least two of the three
lines RP1, RP2, and RP3.
Similarly, Q cannot be on at least two of the three lines RP1,
RP2, and RP3. Hence, at least one
of the lines RP1, RP2, and RP3
has neither P nor Q incident with it. Therefore, there is
a line m with neither P nor Q incident with it.
Since Q is not on line l and
by Case 1, Q is on exactly n + 1 lines m1, m2,
...
, mn+1.
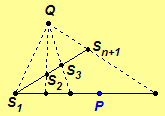 By the Dual of Axiom P1, each line mj
intersects line m at exactly one point Sj for j = 1,
2, ... , n + 1. These n + 1 points
are distinct. For if not, Sj = Sk for some j
≠ k, but then
mj = QSj
= QSk = mk
for j
≠
k, which contradicts that m1, m2,
...
, mn+1 are distinct.
Also, the n + 1 points S1, S2,
...
,Sn+1 are the only
points on m. For if not, there would be another point T on m
distinct from the n +1 points S1, S2,
...
,Sn+1, but then an (n + 2)nd
line QT
≠ mj , j =
1, 2, ... , n + 1, would intersect the
line m, which contradicts that Q is on exactly n + 1
lines. Hence, there are exactly n + 1 points on line m.
Thus P is not on line m and line m contains exactly n
+1 points; therefore, as with Case 1, P is incident with exactly n + 1
lines.
By the Dual of Axiom P1, each line mj
intersects line m at exactly one point Sj for j = 1,
2, ... , n + 1. These n + 1 points
are distinct. For if not, Sj = Sk for some j
≠ k, but then
mj = QSj
= QSk = mk
for j
≠
k, which contradicts that m1, m2,
...
, mn+1 are distinct.
Also, the n + 1 points S1, S2,
...
,Sn+1 are the only
points on m. For if not, there would be another point T on m
distinct from the n +1 points S1, S2,
...
,Sn+1, but then an (n + 2)nd
line QT
≠ mj , j =
1, 2, ... , n + 1, would intersect the
line m, which contradicts that Q is on exactly n + 1
lines. Hence, there are exactly n + 1 points on line m.
Thus P is not on line m and line m contains exactly n
+1 points; therefore, as with Case 1, P is incident with exactly n + 1
lines.
By Cases 1 and 2, every point in a
projective plane of order n is incident with exactly n + 1
lines.//
Theorem P4. In a projective plane of order n, every line is incident with exactly n + 1 points.
A natural question arises, how many points and lines are in a projective plane of order n, which is answered with the next theorem.
Theorem P5. In a projective plane of order n, there exist exactly n2 + n + 1 points and n2 + n + 1 lines.
Proof. Let P be a point in a projective plane of order n, the existence is guaranteed by Axiom P4. Further, points distinct from P exist. By Axiom P1, every point distinct from P must be on exactly one line with P. By Theorem P3, there are exactly n + 1 lines incident with P. By Theorem P4, each of these lines is incident with exactly n points distinct from P. Hence, there are n(n + 1) + 1 = n2 + n + 1 points. By the principle of duality, there are also n2 + n + 1 lines.//
Exercise 1.18. Identify the axioms for a finite projective plane that are valid in Euclidean geometry. Explain why the others are not valid.
Exercise 1.19. Show the axiomatic system for a finite projective plane is incomplete.
Exercise 1.20. Prove Theorem P4.
Exercise 1.21. How many points and lines are in projective planes of order 4, 13, and 27?
I have had my results for a long
time, but I do not yet know how I am to arrive at them.
—![]() Karl Friedrich Gauss (1777–1855)
Karl Friedrich Gauss (1777–1855)
|
© Copyright 2005, 2006 - Timothy Peil |