Fractions, Number Lines, and Order

Finding the Median of a Set of Fractions

Five students brought identical sized pans of bars to sell in a bake sale. The bars were all cut to be the same size. By the end of the sale, three-fourths of Cary's bars, two-thirds of Dana's bars, half of Jamie's bars, five-eighths of Lynn's bars, and seven-eighths of Terry's bars were sold. Whose pan of bars was the median amount sold?

The median of a set of fractions can be found by using the same method that we used for a set of whole numbers. Remember that the median is found by first ordering the set of values, and that a good method for ordering fractional values is to rewrite them with a common denominator.

 

Solution for the above problem: We first find the least common denominator by finding the least common multiple for 4, 3, 2, 6, and 8. We find the LCM by the prime factorization method.

Next, place the fractions in order from least to greatest.

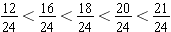
The median value is the 3^rd value of 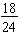. We take the simplest form, so the median is . 

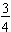We have found that Cary sold the median number of bars.

Example: Find the median of this list:

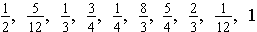
Solution:  First we need to order these values from least to greatest. There are many ways to do this. We could use a number line like the one on the previous page. Here, we will change all of these values to a common denominator:

Now we place these in order from least to greatest and select the median.

Since there are 10 values in the list, we need to find the value that is halfway between the 5^th and 6^th values.  So, the median is . 

 

return to top | previous page | next page