4.4 Desargues' Theorem  Printout
If Desargues, the daring pioneer of the seventeenth century, could have foreseen what his ingenious method of projection was to lead to, he might well have been astonished. He knew that he had done something good, but he probably had no conception of just how good it was to prove.
—E. T. Bell (1883–1960)

        The French mathematician Gérard Desargues (1593–1662) was one of the earliest contributors to the study of synthetic projective geometry. Desargues was an engineer and architect, who had served in the French army. The importance of the theorem, that bears his name, is due to the relating of two aspects of projective geometry: perspectivity from a point and perspectivity from a line. Because of his many contributions to the field of projective geometry, the theorem was named after him even though his major work, Brouillon projet, was lost for nearly two centuries before another French geometer Michel Chasles (1793–1880) discovered a copy in 1845.
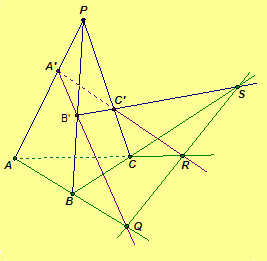        Though we are only studying plane projective geometry, we motivate Desargues' Theorem with a triangular pyramid in three dimensions. The diagram on the left is a triangular pyramid with vertices A, B, C, and P. The triangles ABC and A'B'C' are perspective from the point P. From Euclidean geometry, two nonparallel planes intersect in a line. Therefore, the two planes a  and a' determined by the triangles ABC and A'B'C' intersect in a line l. Since AB and A'B' are in planes a and a', respectively, the point Q = AB · A'B' must be on line l.  Similarly, R = AC · A'C'  and S = BC · B'C' must be on line l. Hence, the triangles ABC and A'B'C' are perspective from the line l = QR.

Axiom 5. (Desargues' Theorem) If two triangles are perspective from a point, then they are perspective from a line.

Click here for a dynamic illustration of Desargues' Theorem GeoGebra or JavaSketchpad.

        In plane projective geometry, Desargues' Theorem cannot be proven from the other axioms; therefore, it is taken as an axiom. The proof of the theorem requires two triangles that are not in the same plane, as illustrated in the motivation example above. That is, Desargues' Theorem can be proven from the other axioms only in a projective geometry of more than two dimensions. Since we have not listed the axioms for a projective geometry in 3-space, we will not discuss the proof of the theorem here, but the proof is similar to the argument made in the illustration above. Many books on projective geometry discuss the topic. (Reference Projective Geometry by Veblen and Young, 1938)

Dual of Desargues' Theorem. If two triangles are perspective from a line, then they are perspective from a point.

Exercise 4.16. Construct two triangles that are perspective from a point, then determine the line from which the triangles are perspective. (You may use dynamic geometry software.)

Exercise 4.17. Construct two triangles that are perspective from a line, then determine the point from which the triangles are perspective. (You may use dynamic geometry software.)

Exercise 4.18. Use dynamic geometry software for this exercise. In the Poincaré Half-plane, construct two triangles that are perspective from a point. Investigate whether the triangles are also perspective from a line.

Exercise 4.19. Given ΔABC and ΔDEF. Assume D is on BC, E is on AC, and F is on AB such that AD, BE, and CF are concurrent. Show that if AB · DE = P, AC · DF = Q, and BC · EF = R, then P, Q, and R are collinear.