TOC & Ch. 0 & Ch. 1 Axiom Table of Contents Ch. 0 Introduction Ch. 1 Axiomatic Systems 1.1.1 Introduction 1.1.2 Examples 1.1.3 History 1.2 A Finite Geometry 1.3 Finite Projective 1.4 Applications

Ch. 2 Neutral Geometry Ch. 2 Table of Contents 2.1.1 Introduction 2.1.2 History 2.1.3 Analytic Models 2.2 Incidence Axioms 2.3 Distance/Ruler Axioms 2.4.1 Plane Separation Axiom 2.4.2 Angle & Measure 2.5.1 Supplement Postulate 2.5.2 SAS Postulate 2.6.1 Parallel Lines 2.6.2 Saccheri Quadrilateral 2.7.1 Euclid Parallel Postulate 2.7.2 Hyperbolic Parallel Postulate 2.7.3 Elliptic Parallel Postulate 2.8 Euclid/Hyperbolic/Elliptic Birkhoff's Axioms Hilbert's Axioms SMSG Axioms

Ch. 3 Transformational Ch. 3 Table of Contents 3.1.1 Introduction 3.1.2 History 3.2.1 Definitions 3.2.2 Analytic Model 3.2.3 Affine Transformation 3.3.1 Isometry 3.3.2 Model/Collinearity 3.3.3 Model/Isometry 3.4.1 Direct Isometry 3.4.2 Model/Direct 3.5.1 Indirect Isometry 3.5.2 Model/Indirect 3.6.1 Similarity Transformation 3.6.2 Model/Similarity 3.7 Other Affine Transformations

Ch. 4 Projective Geometry Ch. 4 Table of Contents 4.1.1 Introduction 4.1.2 Historical 4.2.1 Axioms 4.2.2 Basic Theorems 4.3 Duality 4.4 Desargue's Theorem 4.5.1 Harmonic Sets 4.5.2 Music & Harmonic Sets 4.6.1 Definitions for Projectivity 4.6.2 Fundamental Theorem 4.6.3 Projectivity/Harmonic Sets 4.6.4 Alternate Construction 4.7.1 Conics 4.7.2 Pascal's Theorem 4.7.3 Tangents to Conics

Other Topics Ch. 5 Spherical Geometry Ch. 6 Fractal Geometry Ch. 7 Topology

Appendices Internet Resources Index Geometer's Sketchpad/GeoGebra JavaSketchpad/GeoGebraHTML Video Lectures Logic Review References Acknowledgements

              4.7.2 Pascal’s Theorem  Printout
We are usually convinced more easily by reasons we have found ourselves than by those which have occurred to others.
—Blaise Pascal (1623–1662)

Here, we answer the questions posed at the end of the introductory page on conics in the projective plane. (Any two points may be chosen as the centers of the respective pencils. Do different choices for the centers give different point conics? Do any five points, no three collinear, determine a unique point conic?)

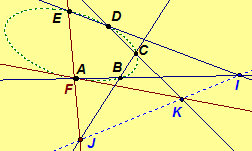
Definition. A simple hexagon ABCDEF is a set of six distinct points A, B, C, D, E, F, no three collinear, called vertices, and the six distinct lines AB, BC, CD, DE, EF, FA, called sides.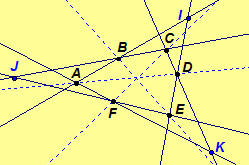 The pairs of points A and D, B and E, and C and F are called opposite vertices. The three pairs of lines determined by opposite vertices are called diagonal lines. The pairs of lines AB and DE, BC and EF, and CD and FA are called opposite sides. The three points of intersection of the opposite sides are called diagonal points.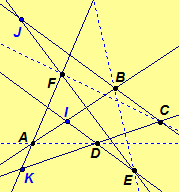

In the figures illustrating a simple hexagon ABCDEF, the points I, J, and K are diagonal points and the dotted lines are diagonal lines. An important note: The order in which the points are listed when naming a simple hexagon is important, since six points do not determine a unique simple hexagon. Further, note how the order and position of the points in the name imply the opposite vertices and opposite sides. Click here for a dynamic illustration of a simple hexagon  GeoGebra or JavaSketchpad.

Exercise 4.46.  (a)  How many ways may the simple hexagon ABCDEF be named?  (b) How many distinct simple hexagons are determined by six distinct points?

Theorem 4.18. A, B, C, D are four points of a point conic defined by projectively related pencils with centers P and P' if and only if the diagonal points of simple hexagon ABCPDP' are collinear.

Proof. Given simple hexagon ABCPDP', let J = 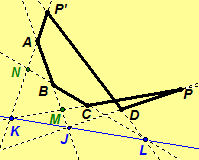PD · AB, K = P'A · PC, and L = P'D · BC be the diagonal points of ABCPDP'. Further, let a = PA, b = PB, c = PC, d = PD, a' = P'A, b' = P'B, c' = P'C, and d' = P'D. We first prove that if a, b, c, d and a', b', c', d' are projectively related pencils with centers P and P', respectively, then J, K, and L are collinear. Click here to investigate with a dynamic illustration GeoGebra or JavaSketchpad.
      Assume A, B, C, D are four points of a point conic defined by projectively related pencils with centers P and P'.  Note  Let M = AB · PC and N = P'A · BC. Note A, J, B, M are collinear and N, L, B, C are collinear; further, J is on d, M is on c, N is on a', and L is on d'. Hence,  and  are elementary correspondences. Thus, we have  Since B is a common element in the projectivity, Corollary 4.12 implies  is a perspectivity. Since A, N, P' are collinear and M, P, C  are collinear, we have AN · MC = P'A · PC = K. Hence, the diagonal point K of the hexagon is the center of the perspectivity, i.e.   Hence, J, L, K are collinear. Therefore, the diagonal points of simple hexagon ABCPDP' are collinear.
      The converse follows by reversing the steps in the argument.//

        Note the positions of P and P' in the name of the simple hexagon ABCPDP' in the theorem. This order is important in using the theorem. We now use Theorem 4.18 to answer the questions posed at the end of the page on conics in the projective plane. That is, do five distinct points determine a unique point conic?

Theorem 4.19. Any five distinct points, no three collinear, determine a unique point conic.

Proof. Let A, B, C, D, and E be five distinct points, no three collinear. By Theorem 4.17, there exists a point conic determined by the projectively related pencils of lines with centers A and B that contains all five points. Let F be a sixth point in this point conic. We assert that F is in the point conic determined when any two of the points A, B, C, D, or E are chosen as the centers of the pencils of lines. We show one case in detail. (How many cases are there?)
       By Theorem 4.18, the diagonal points of simple hexagon DEFACB are collinear, since C, D, E, F are four points of the point conic defined by pencils with centers A and B. Note that DEFACB and EFACBD name the same simple hexagon; therefore, they have the same diagonal points. Hence, by Theorem 4.18, E, F, A, B are points of the point conic defined by pencils with centers C and D. Hence, F is in the point conic defined by C and D.
       Note that in the first line of the preceding paragraph, C, D, and E can be placed in any order. Thus by following the same argument, F is also in the point conics defined by C and E, and D and E. The rest of the cases follow from these cases with various orders of the points A, B, C, D, and E. (Check a some of them.) Therefore, any five distinct points, no three collinear, determine a unique point conic.//

Exercise 4.47. (a)  How many cases are there in the proof of Theorem 4.19?  (b)  Extend the proof to include the case where A and C are the centers.

        An immediate result of Theorems 4.18 and 4.19 is Pascal’s mystic hexagon theorem. Not until after the concept of duality was developed was the dual of Pascal’s Theorem proven.  The dual of Pascal’s Theorem is known Brianchon’s Theorem, since it was proven by C. J. Brianchon (1783–1864) in 1806, over a century after the death of Blaise Pascal. 

Theorem 4.20. (Pascal’s Theorem) If the vertices of a simple hexagon are points of a point conic, then its diagonal points are collinear.

Theorem 4.21. (Brianchon’s Theorem) If the sides of a simple hexagon are lines of a line conic, then the diagonal lines are concurrent.

        Pascal's Theorem may be used to more easily construct points of a point conic.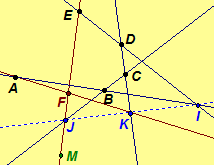 Let A, B, C, D, E be five points, no three collinear. We know these points determine a point conic. Also, any additional point F of the point conic must be a point of the simple hexagon ABCDEF, but we do not have the sides AF or EF. Let M be an arbitrary point to form a line EM (a side of the simple hexagon); then point F will be on line EM. (How do you know such a point M exists?) Then I = AB · DE and J = BC · EM are diagonal points of the hexagon. By Pascal's Theorem, the diagonal points are collinear; therefore, the diagonal point K = CD · IJ. Finally, we locate F by F = EM · AK.
        Click here for a dynamic illustration of a point conic constructed from Pascal's Theorem GeoGebra or JavaSketchpad.

       Use the dynamic geometry construction to investigate what happens when the sixth point, F, constructed using Pascal's Theorem approaches A in the simple hexagon ABCDEF. What appears to be true about the line AK and the point conic? Write your conjecture.

Exercise 4.48. Prove Pascal's Theorem.

Exercise 4.49. Use Pascal's Theorem to construct a sixth point in a point conic formed from five points, no three collinear. (May use dynamic geometry software.)

Exercise 4.50. Use Brianchon's Theorem to construct a sixth line in a line conic formed from five lines, no three concurrent. (May use dynamic geometry software.)