TOC & Ch. 0 & Ch. 1 Axiom Table of Contents Ch. 0 Introduction Ch. 1 Axiomatic Systems 1.1.1 Introduction 1.1.2 Examples 1.1.3 History 1.2 A Finite Geometry 1.3 Finite Projective 1.4 Applications

Ch. 2 Neutral Geometry Ch. 2 Table of Contents 2.1.1 Introduction 2.1.2 History 2.1.3 Analytic Models 2.2 Incidence Axioms 2.3 Distance/Ruler Axioms 2.4.1 Plane Separation Axiom 2.4.2 Angle & Measure 2.5.1 Supplement Postulate 2.5.2 SAS Postulate 2.6.1 Parallel Lines 2.6.2 Saccheri Quadrilateral 2.7.1 Euclid Parallel Postulate 2.7.2 Hyperbolic Parallel Postulate 2.7.3 Elliptic Parallel Postulate 2.8 Euclid/Hyperbolic/Elliptic Birkhoff's Axioms Hilbert's Axioms SMSG Axioms

Ch. 3 Transformational Ch. 3 Table of Contents 3.1.1 Introduction 3.1.2 History 3.2.1 Definitions 3.2.2 Analytic Model 3.2.3 Affine Transformation 3.3.1 Isometry 3.3.2 Model/Collinearity 3.3.3 Model/Isometry 3.4.1 Direct Isometry 3.4.2 Model/Direct 3.5.1 Indirect Isometry 3.5.2 Model/Indirect 3.6.1 Similarity Transformation 3.6.2 Model/Similarity 3.7 Other Affine Transformations

Ch. 4 Projective Geometry Ch. 4 Table of Contents 4.1.1 Introduction 4.1.2 Historical 4.2.1 Axioms 4.2.2 Basic Theorems 4.3 Duality 4.4 Desargue's Theorem 4.5.1 Harmonic Sets 4.5.2 Music & Harmonic Sets 4.6.1 Definitions for Projectivity 4.6.2 Fundamental Theorem 4.6.3 Projectivity/Harmonic Sets 4.6.4 Alternate Construction 4.7.1 Conics 4.7.2 Pascal's Theorem 4.7.3 Tangents to Conics

Other Topics Ch. 5 Spherical Geometry Ch. 6 Fractal Geometry Ch. 7 Topology

Appendices Internet Resources Index Geometer's Sketchpad/GeoGebra JavaSketchpad/GeoGebraHTML Video Lectures Logic Review References Acknowledgements

        2.7.1 Euclidean Parallel Postulate  Printout
This ought even to be struck out of the Postulates altogether; for it is a theorem involving many difficulties.
 —Proclus (410–485)

 

Euclid's Fifth Postulate. That, if a straight line falling on two straight lines make the interior angles on the same side less than two right angles, the two straight lines, if produced indefinitely, meet on that side on which are the angles less than the two right angles.

SMSG Postulate 16. (Euclidean Parallel Postulate) Through a given external point there is at most one line parallel to a given line.

Playfair's Axiom. Through a point not on a line there is exactly one line parallel to the given line.

      Playfair's Axiom is named after John Playfair (1748–1819), a Scottish physicist and mathematician, though many others had used it much earlier. Since we have shown the existence of a parallel line, it is clear that SMSG Postulate 16 (Euclidean Parallel Postulate) and Playfair's Axiom are equivalent. Further, Euclid's Fifth Postulate and the Euclidean Parallel Postulate are equivalent.

Theorem 2.21. In a neutral geometry, Euclid's Fifth Postulate is equivalent to the Euclidean Parallel Postulate.

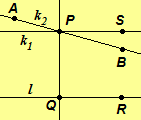Proof. First use Euclid's Fifth Postulate to prove the Euclidean Parallel Postulate. Let l be a line and P be a point not on l. By Theorem 2.12, there is a unique line perpendicular to a given line through a given point; therefore, there is a point Q on l such that line PQ is perpendicular to l. Also, there is a unique line k₁ through P such that line PQ is perpendicular to k₁. By Theorem 2.13, two lines perpendicular to the same line are parallel; therefore, k₁ is parallel to l and P is on k₁.
      We need to show that k₁ is the unique line parallel to l through P. Let k₂ be another line through P such that k₁ and k₂ are distinct lines. Let A and B be distinct points on k₂ such that A-P-B and B and Q are on the same side of k₁. Let R be on l and S be on k₁ such that B, R, and S are all on the same side of line PQ. Hence, since B and S are on the same side of line PQ and B and Q are on the same side of line PS, by the definition of the interior of an angle,  Since line PQ is perpendicular to both l and k₁,  and  are right angles; i.e.  Since   Hence,  Therefore, since B and R are on the same side of line PQ and , by Euclid's Fifth Postulate,  and  intersect on the same side as B and R. Hence, k₁ is the unique line parallel to l that contains P.
      Next, use the Euclidean Parallel Postulate to prove 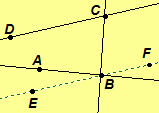Euclid's Fifth Postulate. Given a line BC and two points A and D on the same side of line BC with

 

We need to show that ray BA intersects ray CD. By the Angle Construction Postulate, there is a ray BE with E and A on the same side of line BC such that

 

Let F be a point such that E-B-F, then  and  are a linear pair. Hence  and  are supplementary. Hence,

 

By (2) and (3), . Hence  Since D and F are on opposite sides of line BC,  and  are alternate interior angles. Hence by Theorem 2.15, line EB is parallel to line DC. By (1) and (2),  Hence line AB and line EB are distinct lines through B. Thus, by the Euclidean Parallel Postulate, line AB is not parallel to line DC.
      By Theorem 2.7, since , we have  Thus, since A and C are on the same side of line EB,  and C are on the same side of line EB. Since line EB and line DC are parallel, line DC is on one side of line EB. Hence ray BA intersects line CD.
      Since A and D are on the same side of line BC,  and  are on the same side of line BC. Hence, ray BA intersects ray CD.//

 

      There are many statements that are equivalent to the Euclidean Parallel Postulate, which could be used as the axiom. We list several of them below after the exercises. How many of them can you show are equivalent? The exercises ask you to prove one direction on a few of the statements and to find a counter-example in the Poincaré Half-plane.

Exercises 2.65.  Show the Poincaré Half-plane does not satisfy the Euclidean Parallel Postulate. (a) Use dynamic geometry software to construct an example.   (b) Find an analytic example.

Exercises 2.66.  Show the Poincaré Half-plane does not satisfy Euclid's Fifth Postulate. (a) Use dynamic geometry software to construct an example.   (b) Find an analytic example.

Exercise 2.67. (a) Prove five of the propositions below using the Euclidean Parallel Postulate and Euclid's Fifth Postulate. (Once one proposition has been proven, you may use that proposition in the proof of another.)  (b) Show the Poincaré Half-plane does not satisfy each of the five propositions. (May use dynamic geometry software to construct an example.)

Printout of the following Euclidean Propostions

Euclidean Proposition 2.1. There exists a line and a point not on the line such that there is a unique line through the point that is parallel to the line.

      Note how this proposition differs from Playfair's Axiom. This proposition only says that at least one such point and line exist; whereas, Playfair's Axiom says that it is true for every line and point not on the the line.  The surprising result that in a neutral geometry this proposition implies Playfair's Axiom is called the All or None Theorem. The result is surprising since we only need existence of the parallel property for one line and one point not on the line to know that the parallel property is true everywhere. A proof of the All or None Theorem may be found in either Elementary Geometry from an Advanced Standpoint by Moise, or Geometry: A Metric Approach with Models by Millman and Parker.

Euclidean Proposition 2.2. If A and D are points on the same side of line BC and line BA is parallel to line CD, then

Euclidean Proposition 2.3. If l₁, l₂, l₃ are three distinct lines such that l₁ is parallel to l₂ and l₂ is parallel to l₃, then l₁ is parallel to l₃.

Euclidean Proposition 2.4. If l₁, l₂, l₃ are three distinct lines such that l₁ intersects l₂ and l₂ is parallel to l₃, then l₁ intersects l₃.

Euclidean Proposition 2.5. A line perpendicular to one of two parallel lines is perpendicular to the other.

Euclidean Proposition 2.6. If l₁, l₂, l₃, l₄ are four distinct lines such that l₁ is parallel to l₂, l₃ is perpendicular to l₁, and l₄ is perpendicular to l₂, then l₃ is parallel to l₄.

Euclidean Proposition 2.7. Every two parallel lines have a common perpendicular.

Euclidean Proposition 2.8. The perpendicular bisectors of the sides of a triangle intersect at a point.

Euclidean Proposition 2.9. There exists a circle passing through any three noncollinear points.

Euclidean Proposition 2.10. There exists a point equidistant from any three noncollinear points.

Euclidean Proposition 2.11. A line intersecting and perpendicular to one side of an acute angle intersects the other side.

Euclidean Proposition 2.12. Through any point in the interior of an angle there exists a line intersecting both sides of the angle not at the vertex.

Euclidean Proposition 2.13. If two parallel lines are cut by a transversal, then the alternate interior angles are congruent. (The converse of Theorem 2.15.)

Euclidean Proposition 2.14. The sum of the measures of the angles of any triangle is 180.

Euclidean Proposition 2.15. There exists a triangle such that the sum of the measures of the angles of the triangle is 180.

Euclidean Proposition 2.16. The measure of an exterior angle of a triangle is equal to the sum of the measures of the remote interior angles. (Compare to the Exterior Angle Theorem.)

Euclidean Proposition 2.17. If a point C is not on segment AB but on the circle with diameter AB, then  is a right angle.

Euclidean Proposition 2.18. If  is a right angle, then C is on the circle with diameter AB.

Euclidean Proposition 2.19. The perpendicular bisectors of the legs of a right triangle intersect.

Euclidean Proposition 2.20. There exists an acute angle such that every line intersecting and perpendicular to one side of the angle intersects the other side.

Euclidean Proposition 2.21. There exists an acute angle such that every point in the interior of the angle is on a line intersecting both sides of the angle not at the vertex.

Euclidean Proposition 2.22. If l₁, l₂, l₃, l₄ are four distinct lines such that l₁ is perpendicular to l₂, l₂ is perpendicular to l₃, and l₃ is perpendicular to l₄, then l₁ intersects l₄.

Euclidean Proposition 2.23. There exists a rectangle.

Euclidean Proposition 2.24. There exist two lines equidistant from each other.

Euclidean Proposition 2.25. If three angles of a quadrilateral are right angles, then so is the fourth.

Euclidean Proposition 2.26. There exists a pair of similar triangles that are not congruent. (Two triangles are similar if and only if corresponding angles are congruent and the corresponding sides are proportional.)

Euclidean Proposition 2.27. The diagonals of a Saccheri quadrilateral bisect each other.

Euclidean Proposition 2.28. One of the summit angles of a Saccheri quadrilateral is a right angle.

Euclidean Proposition 2.29. Any three lines have a common transversal.

Euclidean Proposition 2.30. There do not exist three lines such that each two are on the same side of the third.

Euclidean Proposition 2.31. In , if M is the midpoint of segment AB and N is the midpoint of segment AC, then the length of segment MN is equal to half the length of segment BC.